Záznam:
Záznam:
Záznam:
Záznam:
Záznam:

Záznam:
Záznam:
Záznam:
Záznam:
Záznam:

Záznam:
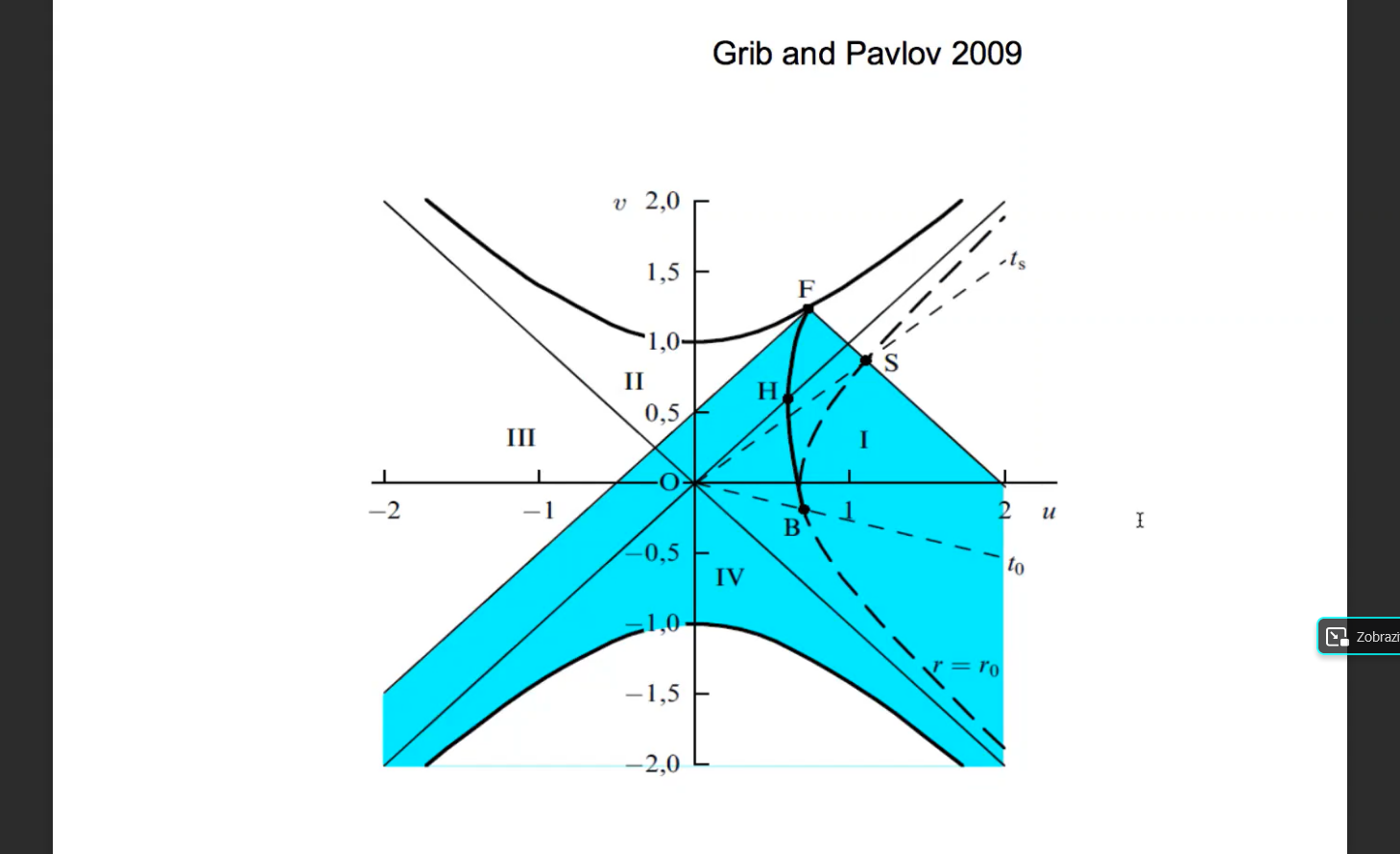
Záznam:

Záznam:
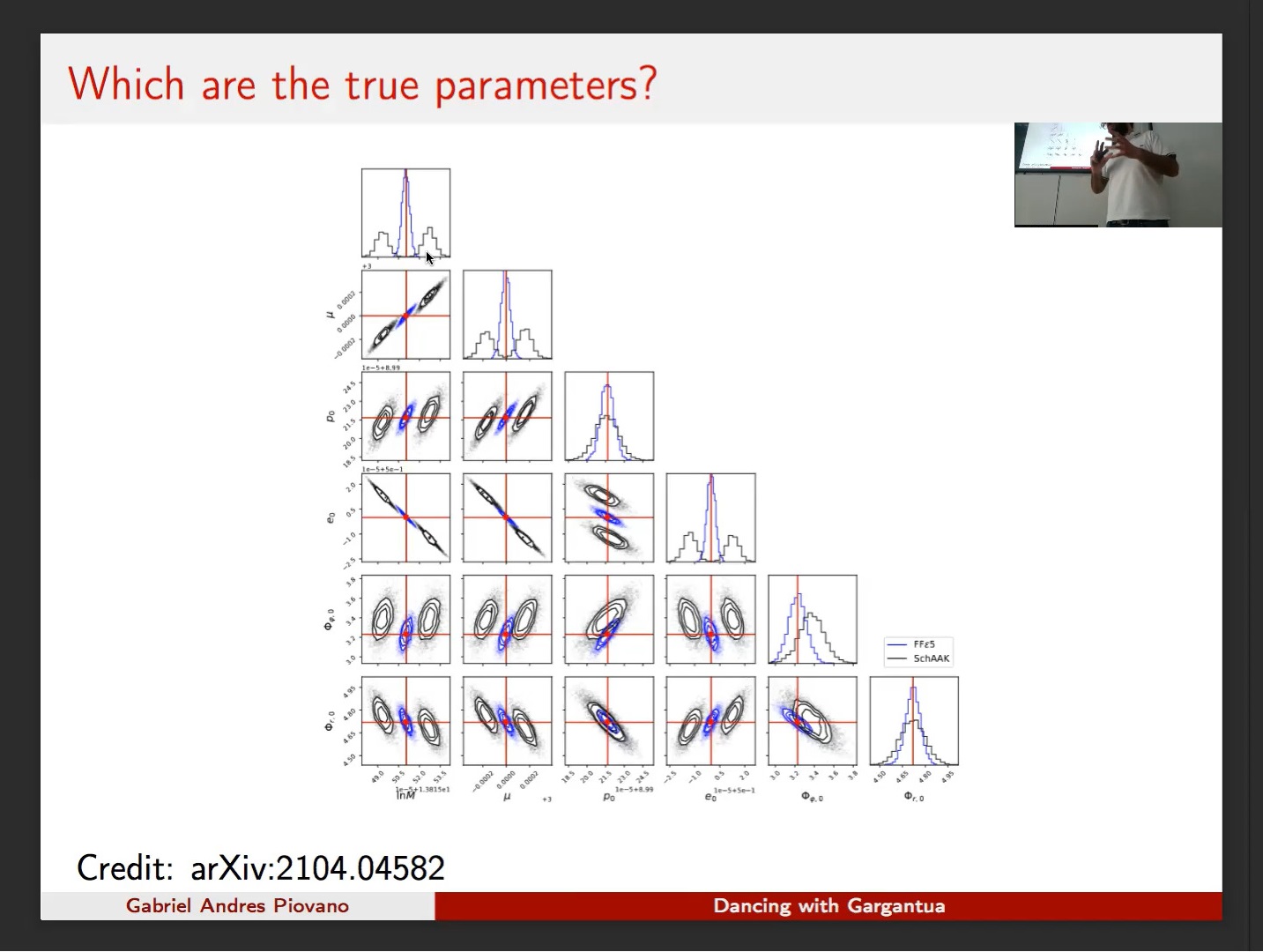
Záznam:
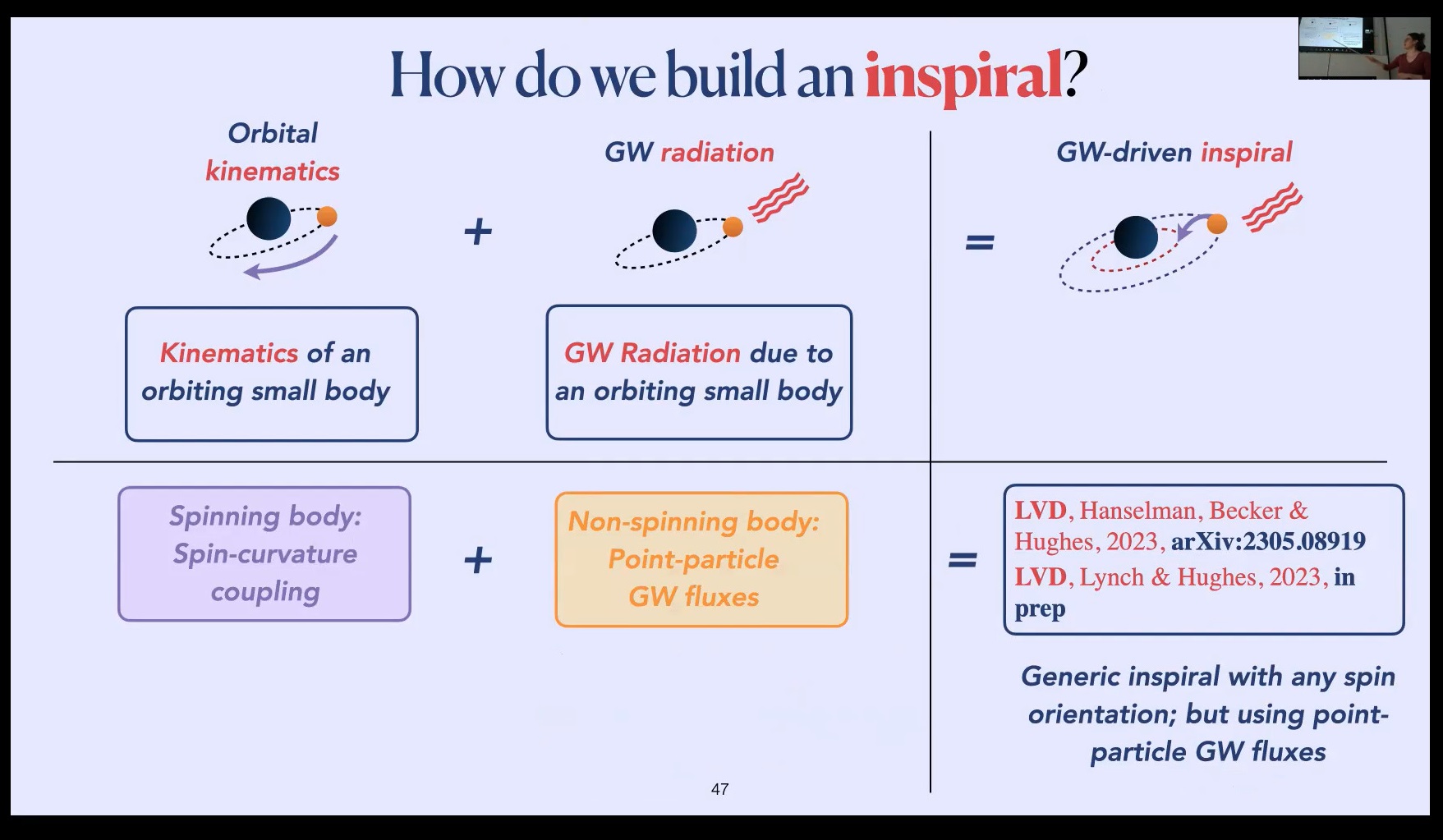
Záznam:
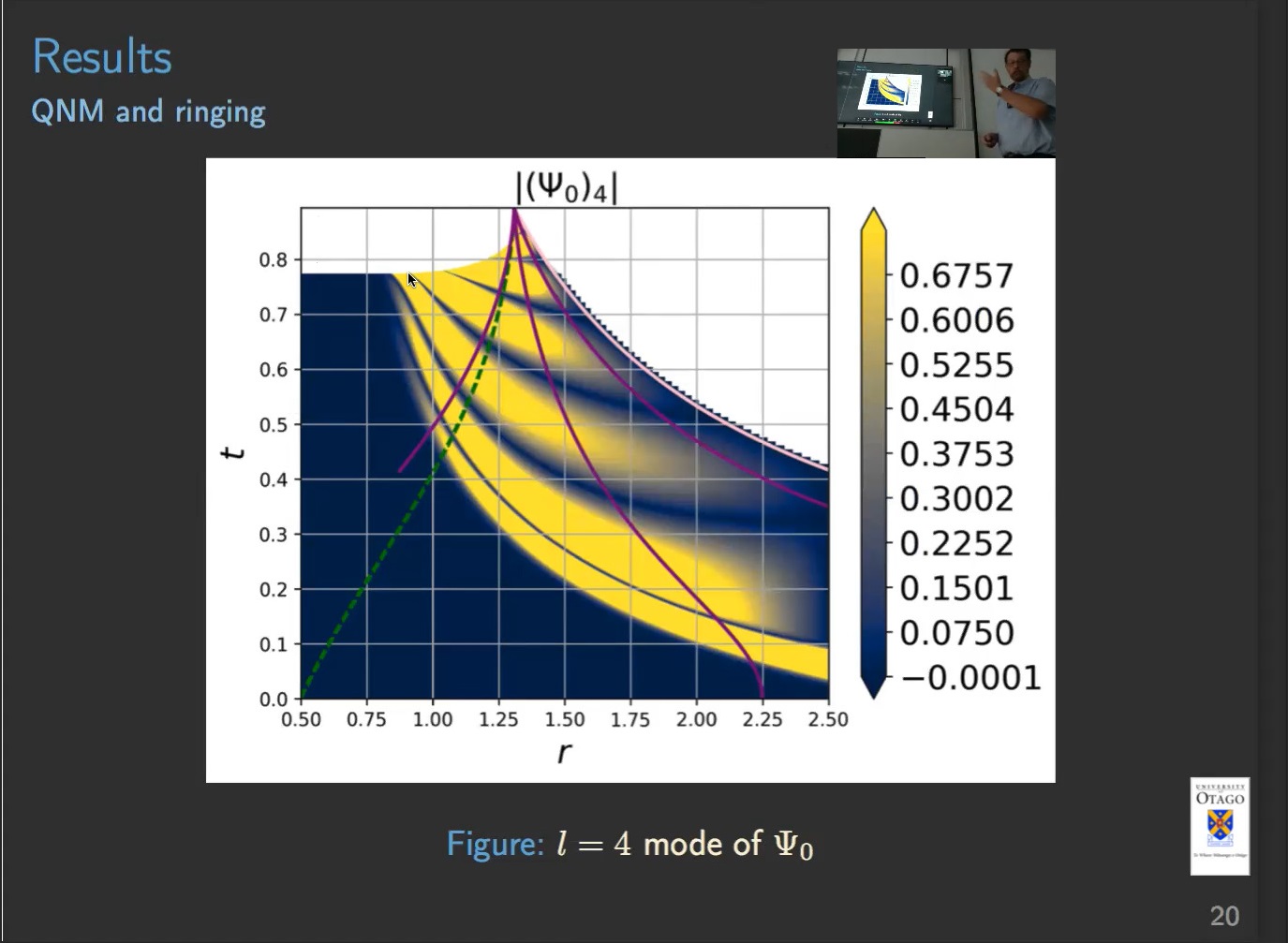
I will discuss a systematic procedure to obtain identities for the derivatives of the metric along a transverse direction on a general null hypersurface of arbitrary dimension. These identities are fully general and could be applied in many contexts of interest, such as degenerate and non-degenerate Killing horizons, homothetical Killing horizons, or even null infinity. In particular, one can establish at which order the obstruction to determine higher order derivatives appears on each case. This is joint work with my PhD supervisor Marc Mars.
Zoom link